|

  
|
||
The Stiles method is restricted to reservoirs with no vertical pressure communication between layers and where the mobility ratio is close to a value of one. The mobility ratio assumption ensures that the velocity of frontal advance of water in each layer remains constant during the flood. That is, the velocities will be different in each layer, as dictated by the following equation, but as the flood progresses the differences between layers will remain constant: ie., there is no velocity dispersion.
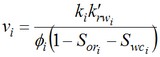
The Stiles procedure is as follows :
| 1. | Inspect the core and log data and divide the section into a total of N separate layers |
| 2. | Order the N layers in the sequence in which they will successively flood-out with water, by applying the above velocity equation. |
| 3. | Generate pseudo-relative permeabilities by applying the following equations. |

|
|
The only difference between Stiles and Heterogeneous VE pseudo-generation is in the flooding order in which the procedures are evaluated.
It is also apparent that, with the exception of gravity within each sand layer, gravity plays no part in the Stiles type displacement on account of the vertical separation of the layers. This results in the displacement efficiency being entirely dictated by the mobility ratio and heterogeneity. Therefore the equation for the fractional flow of water is simplified as follows :
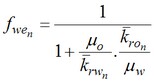
Welge's equation, expressed in hydrocarbon pore volumes, is provided below :

where.
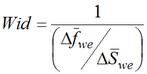
The expression to calculate surface watercut from the reservoir fractional flow value is provided below;
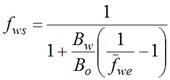
Page url: http://www.YOURSERVER.com/help/index.html?stiles_method.htm